Absolute Maximum Shear Stress Formula

Maximum In-plane Shear Stress Calculator

Maximum In-plane Shear Stresses

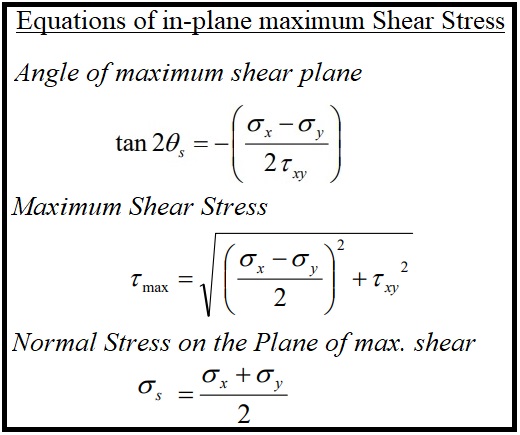
This calculator is for finding maximum and minimum in-airplane shear stress(τ_max and τ_min) and the angles of orientation (θ_max and θ_min) of the planes. It also calculates the average normal stress σ_{due south} acting on the planes of maximum shear stress.
Sign Convention: Positive confront of a plane is that on which the outward normal acts in the positive coordinate direction, otherwise it is negative face. Normal Tensile stresses are taken as positive and normal compressive stresses are negative. Shear stress on a plane is taken as positive if information technology acts in the positive coordinate management on a positive face, or in a negative coordinate direction on a negative face. Otherwise it is taken as negative. Angle of orientation (θ) is -ve for clock-wise and +ve for anti-clockwise rotation with reference to the positive ten-axis

Please note than the maximum and minimum shear stresses are equal in magnitude but contrary in sign. Planes of maximum and minimum shear stress are at xc degrees to each other.

Past default this figurer is using the units of stress as MPa, simply other units N/mm², kN/m², ksi, psi etc. can also be used without affecting the results.

This calculator tin also be used for finding maximum and minimum strain because the equations are same in that case too merely by ignoring the units as strains are dimensionless.

Please refer to the effigy and enter the required values in the course given beneath and so click "Calculate"

The following maximum shear stress Equations are used in this estimator.

maximum shear stress equations